Forums
Blogs
Articles
File Library
FAQ
Search
FB
7
1 votes
Heat Transfer In Buried Liquid Lines (Stagnant Liquid)
13 February 2016
Dear All,My earlier blog entry on heat transfer in buried liquid pipelines related to temperature reduction of the flowing liquid at a certain distance from the pipeline inlet.
Let us think of a buried pipeline or pipeline section where the liquid flow has been stopped and the liquid is now in a stagnant condition in the pipeline or pipeline section. Let us consider that the time at which the pipeline or pipeline section contents are completely at rest (stagnant) is zero i.e, t = 0 seconds. Now let us say that the average temperature of the stagnant liquid at time t = 0 seconds is T0, degrees C. If T0 is higher than the surrounding (soil at pipeline burial depth) temperature than it will start decreasing with time due to heat transfer from the stagnant liquid in the pipeline to the surrounding. We want to know the drop in temperature of the stagnant liquid after a given time period of the stagnant liquid. In other words, we are required to calculate the temperature Tt at a time interval of t = t seconds. The unit seconds are used because the equations require the unit of time as seconds. From a practical point of view a realistic temperature drop will occur in hours or days and time in hours or days needs to be converted into seconds.
Today's blog entry provides the relevant equations for a fair approximation of the temperature drop of a stagnant liquid (no flow) in a buried pipeline. These equations can be used with a fair degree of accuracy for heavy crudes being transported in buried pipelines within the constraints of the value of the empirical constant 'β' selected in the range provided. Let us go to the equations:
where:
TS = soil temperature at pipeline depth, K
Tt, T0 = oil temperature at time t and 0 respectively, K
F = dimensionless 'Fourier' number
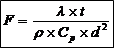
where:
λ = thermal conductivity of liquid, W/(m-K) (for crude oil, λ = 0.13 W/m-K)
t = time, s
ρ = oil or liquid density, kg/m3
Cp = specific heat capacity of liquid, J/(kg-K)
d = pipeline internal diameter, m
β = empirical coefficient (ranges from 6 to 10), depending on heat transfer in the soil around the pipe, the pipe coating, pour point of the liquid and wax layer on pipe wall. A higher value of β means higher thermal conductivity of these layers.
Specific Heat Capacity of Crude Oil
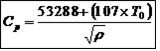
where:
Cp = Specific heat capacity at temperature T0, J/kg-K
T0 = Temperature, ⁰C
ρ = crude oil density at temperature T0, kg/m3
A snapshot of an example calculation in an excel workbook is shown below to give an idea of the temperature drop at time t or in other words the numerical value of Tt.
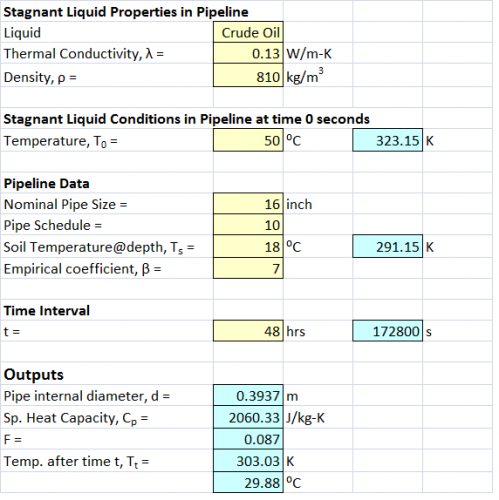
That is all for today. Would be happy to have comments from members of "Cheeresources".
Regards,
Ankur.
Dear Mr. Ankur, would you please quote the references (book / code etc.) to the modelling equations mentioned in your blog-entry?
Thanks ~ opel